The origin of the difference formula is actually quite a funny story. It is simpler than what you would assume it would be. You see the formula comes from this thing called a graph. It is the difference between two points on a graph. It is much simpler than it sounds.
(http://catalog.flatworldknowledge.com/bookhub/reader/4372?e=fwk-redden-ch04_s05)
This picture shows us that the first point is known as x and the first y value for that point is known as f(x) because that is what the value of x is represented by. Then you have a second point that is h distance from x so you name it x+h (the first point plus the distance) and the y value for that one would then be f(x+h) [that is the x values name and since that means value of x you use whatever name we gave it]. Now we have two different points on a graph. With this we can plug our values into the slope formula which is y2-y1/x2-x1. This means if is f(x+h)-f(x)/x+h-x. The xs on the bottom cancel out and you are left with just an h on the denominator. Woah. Look at that. We have a formula that is known as the difference quotient.
On our graph we see that there is also a secant line. The thing is, we want a tangent line so we can use this formula to find the derivatives and specific slopes of that graph. The tangent line only touches the graph once. So the way that we get from secant to tangent is by setting the limit of h as 0. As we approach zero on the graph we get very very very very very very very very very close to the first point that we placed. The two points should overlap.
(http://www.mathcaptain.com/calculus/difference-quotient.html)
In this graph we see that the secant line has turned into a tangent line due to the limit that we gave it (I know these are two different graphs, but the concept remains the same for every graph that you have). The tangent line is then what we use for the rest of the concept. You use it to find the derivative and then the slope formula for the tangent line and then you can find the horizontal tangent and it is just so great. It is not too difficult though. Here is a video to assist you.
(https://www.youtube.com/watch?v=1mlkc3Pfxu4)
References
http://catalog.flatworldknowledge.com/bookhub/reader/4372?e=fwk-redden-ch04_s05
http://www.mathcaptain.com/calculus/difference-quotient.html
https://www.youtube.com/watch?v=1mlkc3Pfxu4
Thursday, June 5, 2014
Monday, May 19, 2014
BQ #6: Unit U- Limits
1. Continuity v. Discontinuity
Continuity is when you can make a graph without taking your pencil off the paper. It is when the graph has no breaks, no jumps, no holes, no oscillating behavior (basically a bridge). The graph is predictable, meaning that the graph goes where you would assume it would go. The limit of the function (discussed later) and the value of the function are the same number. The graph is a smooth, not rigid. The key thing to remember is that just because it changes functions, does not mean that it is not continuous still.
 (http://www.inf.kcl.ac.uk/research/groups/PLANNING/index.php?option=com_content&view=article&id=70&Itemid=77)
(http://www.inf.kcl.ac.uk/research/groups/PLANNING/index.php?option=com_content&view=article&id=70&Itemid=77)
In the picture above, the graph has a lot of odd changes in shape, but it is still a continuous graph. No matter what x value you choose, the limit and the value will be the same number, there are no breaks in the graph, and it can be drawn without taking the pencil off of the paper.
(http://www.mathsisfun.com/calculus/continuity.html)
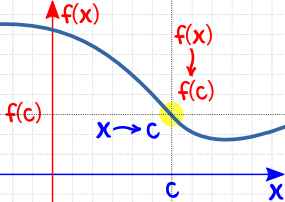
This is also a continuous graph. The values of f(x) (limit) is the same as that of f(c) (value). This is a more a graph that more people are able to associate with more easily. Both this and the previous graph are continuous graphs though. Continuities are seen on a lot of graphs. It is a continual graph, no breaks, predictable, and has the same limit and value.
A discontinuity is what we see as a break, jump, or hole in the graph. These are the graphs that are fragmented and broken. They do not run in a smooth line. They stop abruptly at some points and continue again at either the same x value in a different spot or at another part on the graph. We have two different families of discontinuities. The first family is known as a removable discontinuity. They are removable for specific reasons (discussed in part 3). The only removable discontinuity that we have is known as a point discontinuity.This looks like a hole in the graph.

(http://www.tutorvista.com/content/math/discontinuity/)
This is a point discontinuity because it creates a hole in your graph. It has a limit, but it does not have a defined value. The value is undefined because there is something in the function that prevents the graph from reaching that point on the graph. The limit is where that hole is though because it was the spot that the graph intended to reach.

(http://hardycalculus.com/calcindex/IE_discontinuity.htm)
This is also a point discontinuity because it has the break in the graph. However, unlike the one that we looked at above, this one has a value (the darker red dot). The limit is the spot on the graph where the hole exists (the intended height of that x value in that spot) and the value is the red dot because that is the actual value of the function at that x-value. Both are examples of removable discontinuities (can mathematically make the function work).
There are also other discontinuities that are known as non-removable discontinuities because they cannot be changed. There are three types of discontinuities: jump, infinite, and oscillating behavior.
The first is a jump discontinuity. The graph breaks at one point and either continues below or above that spot in the same x position. The graph can either have to open circles or one open and one closed. An open circle means that the graph cannot touch that value and a closed circle means the graph touches that value (hence why we cannot have two open circles). The limit does not exist here because the graph has two different values for the same x value (different left/right values). The graph looks like this:

(http://en.wikibooks.org/wiki/Calculus/Continuity)
As you can tell, the value of 0 has a left value of -1 and a right value of 1, creating a jump in the graph and therefore making it a discontinuity. The limit does not exist, but the value does.
Another discontinuity is the infinite discontinuity. It gets its name because as it heads to a certain point on the graph, it turns towards either infinite of negative infinite and creates a vertical asymptote in the graph ( a spot where the graph will head to that point, but never actually touch it). This creates unbounded behavior on the graph making a break in the graph, denying the value of x to have a limit or a value, and making it a discontinuous graph. It looks like this:

(http://shell.cas.usf.edu/~ssuen/2233.2013s/c/chapter1.diagrams/images01.html)
The graph is discontinuous because it has the infinite discontinuity at -3 creating the vertical asymptote and unbounded behavior.
The third and final discontinuity is known as oscillating behavior. This is when in a very small portion of the graph, it creates a large amount of closely knit wiggly lines. It is a discontinuity because the graph is never intending to go to a certain point for that x-value. It is just wiggly. It creates an untraceable line and does not have a limit or a value. It cannot be fixed.

(http://en.wikipedia.org/wiki/Classification_of_discontinuities)
This an example of oscillating behavior because prior to the point on the graph where it hits zero on the y-axis, the graph just wiggles. It creates so many up and down portions that they blur together and make it look like a solid mass on the graph. It does not intend to go anywhere and it contains no value.
In the end, continuity is when the graph has no breaks, no jumps, no holes, is predictable, has the same number for the limit and value, and can be drawn without taking your pencil off of the paper. A discontinuity is a break, a jump, a hole, a non-matching limit and value, not predictable, and cannot be drawn without taking the pencil off of the paper.
2. Limit or Value?
A limit is the intended height of a graph, the point that it meant to go to, but might not have actually "reached". The limit only exists for removable discontinuities and for continuous graphs. This is because the graph actually reaches a point on the graph. The jump, infinite, and oscillating behavior discontinuities do not have a limit because they never touch an intended point or they have two y-values for a single x-value that will never connect with one another, or because it just wiggles too much in too small of an area. The limit is reached only for a point discontinuity because if you trace the graph from both sides to that point, you get to a single point. With all the other points, no single point is reached meaning there was no intended height and there was no limit.

(http://math.stackexchange.com/questions/137609/proving-the-non-existence-of-a-limit)
This is an example of when the limit does not exist. The left and right limit statements come to two different values and the graph does not meet at a single intended height meaning that the limit does not exist. However, the value does exist. The values is the filled in circle and that is at 1. See the difference there?
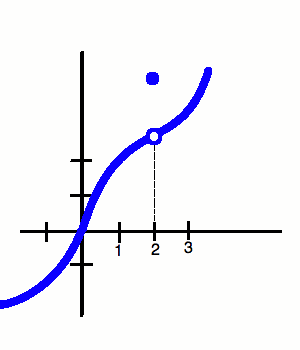
(http://www.wyzant.com/resources/lessons/math/calculus/limits)
Here the limit and the value both exist. The limit exists because the graph touches in the same spot (the left and right limit statements are the same). The graph meets at 3 when the x-value is 2. That is the limit, the intended height. The actual value is the darker circle which seems to be visible at 5. This is an example when both exist. For a continuous graph the limit and the value will be the same for the whole entire graph.
3. A.N.G.
Do not fear. This is not some kind of gang. This is the three letters from the word VANG that we use for limits: Algebraically Numerically and Graphically.
Algebraically is when we use either direct substitution, factoring out/dividing method, rationalizing/conjugate method, or limit infinity. The video below has some spots where it is blurry, but the examples are there for the first three methods to help you get the basic understanding of each type. Remember you always want to use direct substitution first!
(https://www.youtube.com/watch?v=8z4aofW85K4)
This next video explains how to algebraically solve one dealing with infinity. Do not fear it is simpler than it seems. The video explains things a little bit differently than learned in class, but the idea and the shortcut are there.
(https://www.youtube.com/watch?v=75xO9xy7TTQ)
Numerically: This is when we use a table to find the values of the function as you get closer and closer to the
number. It helps you find what the limit should be and then when you solve for it you see if the limit actually exists. You should usually take the number being approached and add and subtract 1/10 and move closer in two on either side. In the video he uses 1/100. As long as you are getting extremely close to the number it is okay.
Graphically: This is when you take your fingers, trace the graph to the point you want to get to and see what the limit is. From there you can see if the limit is present or if it does not exist. If it does not exist, you write one sided limit statements to help you understand where each part (the left and the right) pieces of the graphs are going. It is not too difficult and is pretty simple to understand.
(https://www.youtube.com/watch?v=IEfoQeWm8Fc)
Resources
http://www.inf.kcl.ac.uk/research/groups/PLANNING/index.php?option=com_content&view=article&id=70&Itemid=77
http://www.mathsisfun.com/calculus/continuity.html
http://www.tutorvista.com/content/math/discontinuity/
http://hardycalculus.com/calcindex/IE_discontinuity.htm
http://en.wikibooks.org/wiki/Calculus/Continuity
http://shell.cas.usf.edu/~ssuen/2233.2013s/c/chapter1.diagrams/images01.html
http://en.wikipedia.org/wiki/Classification_of_discontinuities
http://math.stackexchange.com/questions/137609/proving-the-non-existence-of-a-limit
http://www.wyzant.com/resources/lessons/math/calculus/limits
https://www.youtube.com/watch?v=75xO9xy7TTQ
https://www.youtube.com/watch?v=8z4aofW85K4
https://www.youtube.com/watch?v=IEfoQeWm8Fc
Continuity is when you can make a graph without taking your pencil off the paper. It is when the graph has no breaks, no jumps, no holes, no oscillating behavior (basically a bridge). The graph is predictable, meaning that the graph goes where you would assume it would go. The limit of the function (discussed later) and the value of the function are the same number. The graph is a smooth, not rigid. The key thing to remember is that just because it changes functions, does not mean that it is not continuous still.
 (http://www.inf.kcl.ac.uk/research/groups/PLANNING/index.php?option=com_content&view=article&id=70&Itemid=77)
(http://www.inf.kcl.ac.uk/research/groups/PLANNING/index.php?option=com_content&view=article&id=70&Itemid=77)In the picture above, the graph has a lot of odd changes in shape, but it is still a continuous graph. No matter what x value you choose, the limit and the value will be the same number, there are no breaks in the graph, and it can be drawn without taking the pencil off of the paper.

(http://www.mathsisfun.com/calculus/continuity.html)
This is also a continuous graph. The values of f(x) (limit) is the same as that of f(c) (value). This is a more a graph that more people are able to associate with more easily. Both this and the previous graph are continuous graphs though. Continuities are seen on a lot of graphs. It is a continual graph, no breaks, predictable, and has the same limit and value.
A discontinuity is what we see as a break, jump, or hole in the graph. These are the graphs that are fragmented and broken. They do not run in a smooth line. They stop abruptly at some points and continue again at either the same x value in a different spot or at another part on the graph. We have two different families of discontinuities. The first family is known as a removable discontinuity. They are removable for specific reasons (discussed in part 3). The only removable discontinuity that we have is known as a point discontinuity.This looks like a hole in the graph.

(http://www.tutorvista.com/content/math/discontinuity/)
This is a point discontinuity because it creates a hole in your graph. It has a limit, but it does not have a defined value. The value is undefined because there is something in the function that prevents the graph from reaching that point on the graph. The limit is where that hole is though because it was the spot that the graph intended to reach.

(http://hardycalculus.com/calcindex/IE_discontinuity.htm)
This is also a point discontinuity because it has the break in the graph. However, unlike the one that we looked at above, this one has a value (the darker red dot). The limit is the spot on the graph where the hole exists (the intended height of that x value in that spot) and the value is the red dot because that is the actual value of the function at that x-value. Both are examples of removable discontinuities (can mathematically make the function work).
There are also other discontinuities that are known as non-removable discontinuities because they cannot be changed. There are three types of discontinuities: jump, infinite, and oscillating behavior.
The first is a jump discontinuity. The graph breaks at one point and either continues below or above that spot in the same x position. The graph can either have to open circles or one open and one closed. An open circle means that the graph cannot touch that value and a closed circle means the graph touches that value (hence why we cannot have two open circles). The limit does not exist here because the graph has two different values for the same x value (different left/right values). The graph looks like this:

(http://en.wikibooks.org/wiki/Calculus/Continuity)
As you can tell, the value of 0 has a left value of -1 and a right value of 1, creating a jump in the graph and therefore making it a discontinuity. The limit does not exist, but the value does.
Another discontinuity is the infinite discontinuity. It gets its name because as it heads to a certain point on the graph, it turns towards either infinite of negative infinite and creates a vertical asymptote in the graph ( a spot where the graph will head to that point, but never actually touch it). This creates unbounded behavior on the graph making a break in the graph, denying the value of x to have a limit or a value, and making it a discontinuous graph. It looks like this:

(http://shell.cas.usf.edu/~ssuen/2233.2013s/c/chapter1.diagrams/images01.html)
The graph is discontinuous because it has the infinite discontinuity at -3 creating the vertical asymptote and unbounded behavior.
The third and final discontinuity is known as oscillating behavior. This is when in a very small portion of the graph, it creates a large amount of closely knit wiggly lines. It is a discontinuity because the graph is never intending to go to a certain point for that x-value. It is just wiggly. It creates an untraceable line and does not have a limit or a value. It cannot be fixed.
(http://en.wikipedia.org/wiki/Classification_of_discontinuities)
This an example of oscillating behavior because prior to the point on the graph where it hits zero on the y-axis, the graph just wiggles. It creates so many up and down portions that they blur together and make it look like a solid mass on the graph. It does not intend to go anywhere and it contains no value.
In the end, continuity is when the graph has no breaks, no jumps, no holes, is predictable, has the same number for the limit and value, and can be drawn without taking your pencil off of the paper. A discontinuity is a break, a jump, a hole, a non-matching limit and value, not predictable, and cannot be drawn without taking the pencil off of the paper.
2. Limit or Value?
A limit is the intended height of a graph, the point that it meant to go to, but might not have actually "reached". The limit only exists for removable discontinuities and for continuous graphs. This is because the graph actually reaches a point on the graph. The jump, infinite, and oscillating behavior discontinuities do not have a limit because they never touch an intended point or they have two y-values for a single x-value that will never connect with one another, or because it just wiggles too much in too small of an area. The limit is reached only for a point discontinuity because if you trace the graph from both sides to that point, you get to a single point. With all the other points, no single point is reached meaning there was no intended height and there was no limit.
(http://math.stackexchange.com/questions/137609/proving-the-non-existence-of-a-limit)
This is an example of when the limit does not exist. The left and right limit statements come to two different values and the graph does not meet at a single intended height meaning that the limit does not exist. However, the value does exist. The values is the filled in circle and that is at 1. See the difference there?

(http://www.wyzant.com/resources/lessons/math/calculus/limits)
Here the limit and the value both exist. The limit exists because the graph touches in the same spot (the left and right limit statements are the same). The graph meets at 3 when the x-value is 2. That is the limit, the intended height. The actual value is the darker circle which seems to be visible at 5. This is an example when both exist. For a continuous graph the limit and the value will be the same for the whole entire graph.
3. A.N.G.
Do not fear. This is not some kind of gang. This is the three letters from the word VANG that we use for limits: Algebraically Numerically and Graphically.
Algebraically is when we use either direct substitution, factoring out/dividing method, rationalizing/conjugate method, or limit infinity. The video below has some spots where it is blurry, but the examples are there for the first three methods to help you get the basic understanding of each type. Remember you always want to use direct substitution first!
(https://www.youtube.com/watch?v=8z4aofW85K4)
This next video explains how to algebraically solve one dealing with infinity. Do not fear it is simpler than it seems. The video explains things a little bit differently than learned in class, but the idea and the shortcut are there.
(https://www.youtube.com/watch?v=75xO9xy7TTQ)
Numerically: This is when we use a table to find the values of the function as you get closer and closer to the
number. It helps you find what the limit should be and then when you solve for it you see if the limit actually exists. You should usually take the number being approached and add and subtract 1/10 and move closer in two on either side. In the video he uses 1/100. As long as you are getting extremely close to the number it is okay.
Graphically: This is when you take your fingers, trace the graph to the point you want to get to and see what the limit is. From there you can see if the limit is present or if it does not exist. If it does not exist, you write one sided limit statements to help you understand where each part (the left and the right) pieces of the graphs are going. It is not too difficult and is pretty simple to understand.
(https://www.youtube.com/watch?v=IEfoQeWm8Fc)
Resources
http://www.inf.kcl.ac.uk/research/groups/PLANNING/index.php?option=com_content&view=article&id=70&Itemid=77
http://www.mathsisfun.com/calculus/continuity.html
http://www.tutorvista.com/content/math/discontinuity/
http://hardycalculus.com/calcindex/IE_discontinuity.htm
http://en.wikibooks.org/wiki/Calculus/Continuity
http://shell.cas.usf.edu/~ssuen/2233.2013s/c/chapter1.diagrams/images01.html
http://en.wikipedia.org/wiki/Classification_of_discontinuities
http://math.stackexchange.com/questions/137609/proving-the-non-existence-of-a-limit
http://www.wyzant.com/resources/lessons/math/calculus/limits
https://www.youtube.com/watch?v=75xO9xy7TTQ
https://www.youtube.com/watch?v=8z4aofW85K4
https://www.youtube.com/watch?v=IEfoQeWm8Fc
Monday, April 21, 2014
BQ#4 – Unit T Concept 3
Uphill or Downhill?
In the image above we see the different quadrants and which ratios are positive in each quadrant and which ones are negative. When we look at tangent and cotangent (since they are the inverse of one another) we see that they are positive in the same quadrants and negative in the same quadrant. The only difference they will see when we begin to graph them is the asymptotes (the areas where they encounter an undefined ratio). This difference changes the shape of the graph slightly. The asymptotes will dominate and be the reason they take a
different direction.
This photo shows the different quadrants on the graph though. We see quadrant one as red, two as green, three as orange, and fourth as blue. The red is positive (+,+), the green is negative (-,+), the orange is positive (-,-), and the blue is negative (+,-). This is for the tangent and cotangent graph though. Other graphs and ratios have other variations. Tangent
When we look at the tangent graph we have to remember that the ratio. That the ratio for a tangent is sine over cosine. That means that whenever cosine (x) is zero, there will be an undefined term. This results in an asymptote. That means that in one period there will be two asymptotes that will need to be avoided. Those are at pi/2 and 3pi/2. (Look at the image below)
.png)
Then we start to place the graph. We know that tangent cannot touch the asymptote at pi/2 and must be above the x-axis in the red rectangle so that means that we make the curve from the zero up and towards the asymptote. That is because pi/2 is the ratio of 1/0 (90 degrees) and that is an undefined term. (Below)
(https://www.desmos.com/calculator/cwdr1eyszr)
After that we move past the asymptote and go to the second quadrant (the green) which is below the x-axis (negative). This piece of the graph cannot touch the spot of pi/2 because that is an asymptote, but it can cross though pi so it will start near the asymptote and cross the x-axis at pi and turn into a positive in the orange section (the third quadrant). The reason pi is not an asymptote is because sine is 0 and cosine is -1 which makes it a zero.
(https://www.desmos.com/calculator/cwdr1eyszr)
As it turns up into the third quadrant it becomes positive. It must avoid the asymptote at 3pi/2 because that is another undefined term (-1/0) and that means it can get close, but never touch it. That is why the graph goes up. It is positive and near the asymptote.
(https://www.desmos.com/calculator/cwdr1eyszr)
Finally the fourth quadrant (the blue) is negative so the graph has to start down below the x-axis again. It must avoid the asymptote at 3pi/2, but because 2pi is not an undefined term (0/1) it can be crossed through so the graph will turn in the upwards direction once hitting that spot.
(https://www.desmos.com/calculator/cwdr1eyszr)
Overall when we are looking at the tangent graph and the asymptotes that are created by the unit circle and knowing that the quadrants dictate the location that the graph has to have we can see why the graph for a tangent graph is uphill. The graph starts at positive and goes negative to positive again, but because the asymptotes exist at the pi/2 and 3pi/2 (where cosine is zero) the graph has to adjust direction to make it work. The middle two sections is one period. The connected halves starting at the bottom and going positive, lying between two asymptotes is the clearest picture of the real reason the graph goes up. The graph cannot touch either asymptote and has to cross at pi, but remain in the proper areas making it go in the uphill direction.
Cotangent
When we are looking at cotangent we have to remember that the ratio has changed, not the quadrant areas, but the ratios (cosine/sine). This change means that the asymptotes has also changed. When the asymptotes change, the direction of the graph must change to make sure that everything works as it is supposed to. So the colors and the labels for the graph remain the same as the tangent graph, but now it has three asymptotes. These undefined areas are at 0, pi, and 2pi (locations on the unit circle where y is equal to zero making the ratio 1/0 or -1/0).
(https://www.desmos.com/calculator/cwdr1eyszr)
Then we begin to graph. The first asymptote is at zero and the first quadrant is positive. That means that it must not cross the zero mark on the graph. That means it must start up and go through the x-axis in a downwards direction. It will cross the x-axis at pi/2 and then cross into the green as a negative. This means it will have to go down.
(https://www.desmos.com/calculator/cwdr1eyszr)
As it goes into the green it turns negative and must avoid the next asymptote at pi. This is again because the ratio is an undefined term (-1/0 deemed by the unit circle). The graph goes through pi and turns negative and does not touch the asymptote at pi. It makes the graph look like a downhill graph.
(https://www.desmos.com/calculator/cwdr1eyszr)
The third part of the graph must be positive again in the orange quadrant because the ratio is two negatives. It must also avoid the asymptote at pi so it starts in the positive region at the turns down at the 3pi/2. That point on the x-axis is where the graph turns negative again. It flips and then attempts to avoid the third asymptote in the first period.
(https://www.desmos.com/calculator/cwdr1eyszr)
For the final area of the graph we see that it is negative again (the ratio makes this) and it cannot touch 2pi (the undefined). It makes the two periods of the tangent graph. It is the final point in the two cycles.
(https://www.desmos.com/calculator/cwdr1eyszr)As an overall idea, the cotangent graph goes downhill because it is avoiding the asymptotes that are created and stay within the positive and negative areas of the graph. It is different than the tangent graph because the asymptotes are different due to the differed ratios.
References
Mrs. Kirch on https://www.desmos.com/calculator/cwdr1eyszr
http://www.pinkmonkey.com/studyguides/subjects/calc/chap1/c0101601.asp
Friday, April 18, 2014
BQ#3-Unit T Concepts 1-3
(https://www.desmos.com/calculator/hjts26gwst)
In the picture above we see a sine graph (the red one) and a cosine graph (the green one). There is also four regions shaded. The red is the first quadrant according to the unit circle, the green the second quadrant, the orange the third quadrant, and the blue the fourth quadrant. Both graphs follow the unit circle of positive where they need to be and negative where they need to be. Remember that sine goes positive positive then negative negative and takes 2pi to repeat and cosine is positive negative negative positive and also takes 2pi to restart. They abide by the rules of being greater than negative one and less than one. It is the basic graph.
Asymptotes
In the picture above we see a sine graph (the red one) and a cosine graph (the green one). There is also four regions shaded. The red is the first quadrant according to the unit circle, the green the second quadrant, the orange the third quadrant, and the blue the fourth quadrant. Both graphs follow the unit circle of positive where they need to be and negative where they need to be. Remember that sine goes positive positive then negative negative and takes 2pi to repeat and cosine is positive negative negative positive and also takes 2pi to restart. They abide by the rules of being greater than negative one and less than one. It is the basic graph.
Asymptotes
Now when we look at the graph we see that there are five different dotted lines and those are all places that asymptotes can exist depending on the trig function that you are using. The first, third, and fifth asymptotes exist only when we have a cosecant and cotangent graph because their ratios have y in the bottom and when y is zero then the ratio becomes undefined and we have a whole. The second and fourth are asymptotes that are seen when we have tangent and secant graphs because the ratio places x in the bottom and those are the spots where x is zero making it an undefined value.
Tangent
Since the asymptotes exist at pi/2 and 3pi/2 the graph needs to avoid these spots on the graph. These asymptotes are also the spots where cosine cross the x-axis at zero. This is because the tangent ratio is sine/cosine and on the unit circle cosine is zero at those two locations. The same apply to the graph.
(https://www.desmos.com/calculator/hjts26gwst)
In this photo we have the first quadrant of the tangent graph. It needs to stay positive for the first quadrant and avoid the asymptote so it goes uphill and does not touch the line of pi/2.
(https://www.desmos.com/calculator/hjts26gwst)
Here we can see that the second part of the graph is drawn. It needs to be negative and it also needs to avoid the asymptote of pi/2. In order to make this possible it goes below the x-axis. This is fair to the ratios on the unit circle because one is negative and one is positive.
(https://www.desmos.com/calculator/hjts26gwst)
The next part of the graph is the piece from the third quadrant. This continues from the second quadrant and pulls into the positive because that is what tangent ratios appear as on the unit circle. It can continue from the previous portion of the graph because there is no asymptote at pi because the unit circle ratios here are 1/1 and that is a number that exists and can be passed through. It does however have to avoid the asymptote that is created by the sine being 1 and the cosine being 0, creating an undefined term.
(https://www.desmos.com/calculator/hjts26gwst)
Tangent
Since the asymptotes exist at pi/2 and 3pi/2 the graph needs to avoid these spots on the graph. These asymptotes are also the spots where cosine cross the x-axis at zero. This is because the tangent ratio is sine/cosine and on the unit circle cosine is zero at those two locations. The same apply to the graph.
(https://www.desmos.com/calculator/hjts26gwst)
In this photo we have the first quadrant of the tangent graph. It needs to stay positive for the first quadrant and avoid the asymptote so it goes uphill and does not touch the line of pi/2.
(https://www.desmos.com/calculator/hjts26gwst)
Here we can see that the second part of the graph is drawn. It needs to be negative and it also needs to avoid the asymptote of pi/2. In order to make this possible it goes below the x-axis. This is fair to the ratios on the unit circle because one is negative and one is positive.
(https://www.desmos.com/calculator/hjts26gwst)
The next part of the graph is the piece from the third quadrant. This continues from the second quadrant and pulls into the positive because that is what tangent ratios appear as on the unit circle. It can continue from the previous portion of the graph because there is no asymptote at pi because the unit circle ratios here are 1/1 and that is a number that exists and can be passed through. It does however have to avoid the asymptote that is created by the sine being 1 and the cosine being 0, creating an undefined term.
(https://www.desmos.com/calculator/hjts26gwst)
This is the final part of one period of the graph. This means that the graph can start over again. The negative has been hit again. The fourth quadrant on the graph is a negative because it is a negative number over a positive number under. This means that it goes under the x-axis and turns up at the x-axis because no asymptote exists at 2pi for tangent graphs. It does however, have to make sure to avoid the asymptote at 3pi/2 (the same reason as the last portion of the graph). That is really all to the tangent graph. It will go like this on and on.
Cotangent
For the cotangent graphs there are three asymptotes within one revolution of the unit circle. These exist because they are the three locations on the unit circle that the ratio has a zero in the bottom (as the sine value). When you divide something by zero we have something known as an asymptote. The graph must stay in the positive and negative regions-according to the unit circle-and avoid the asymptotes. These asymptotes exist wherever sine touches the x-axis at zero because the asymptotes are created by sine being zero and making it undefined.
.png)
.png)
For the cotangent graphs there are three asymptotes within one revolution of the unit circle. These exist because they are the three locations on the unit circle that the ratio has a zero in the bottom (as the sine value). When you divide something by zero we have something known as an asymptote. The graph must stay in the positive and negative regions-according to the unit circle-and avoid the asymptotes. These asymptotes exist wherever sine touches the x-axis at zero because the asymptotes are created by sine being zero and making it undefined.
.png)
The first part of the graph lies in the upper portion of the graph because on the unit circle it is positive due to both sine and cosine being positive values. It starts at the top, avoiding contact with the zero asymptote (0 degrees) and then going negative after the crossing of the x-axis. It crosses the axis at the same point that the cosine touches the x axis.
For the second part of the graph it continues from the first part and goes negative because cosine is negative and sine is positive. This portion also has to avoid the asymptote at pi (created because sine has a zero value making it undefined). This creates one whole period of the cotangent graph.
.png)
The third part of the graph is again above the x-axis because sine and cosine are both negative making it a positive. It must avoid the asymptote on its left so it gets close, but just like the previous portion it cannot touch it. Then it heads down and passes through the x-axis at the same point that cosine passes through the x-axis.
The fourth part of the graph is continued from the previous portion. It is negative because sine is negative and cosine is positive. Then it goes only as far as the asymptote that is created by the sine being zero on the unit circle It cannot touch that one. Now we have the full two periods of the cotangent graph.
Cosecant
This is the inverse function of sine. It means that it will have asymptotes that are the same as cotangent because cosecant, like cotangent, has the sine on the bottom and will have the zeros occur in the same spots. It will follow the same pattern as the sine graph of positive positive and negative negative to have a 2pi period, but it does have its differences. It needs the sine graph though so that the real graph can be created.
This is the inverse function of sine. It means that it will have asymptotes that are the same as cotangent because cosecant, like cotangent, has the sine on the bottom and will have the zeros occur in the same spots. It will follow the same pattern as the sine graph of positive positive and negative negative to have a 2pi period, but it does have its differences. It needs the sine graph though so that the real graph can be created.
(https://www.desmos.com/calculator/hjts26gwst)
In this picture the first quadrant is shown with the first part of the cosecant graph. It is avoid the asymptote because that is an undefined spot as we discussed in cotangent graphs. It is starting from the maximum of the sine graph and only displaying one half of it. It is there because the ratios on the unit circle dictate that it is positive and that it must avoid the zero asymptote. This is one portion of the graph though. Between the asymptotes there are two halves. the other half lies in the second quadrant.
(https://www.desmos.com/calculator/hjts26gwst)
In the second quadrant we see a full parabola. The graph remains positive still because the unit circle dictates that it will remain positive. The second portion of the parabola starts at the maximum of the sine graph as well. Now we have a positive parabola that originates off of sine's maximum and avoids the two quadrant angles that create an undefined value (the asymptotes).
(https://www.desmos.com/calculator/hjts26gwst)In this picture the first quadrant is shown with the first part of the cosecant graph. It is avoid the asymptote because that is an undefined spot as we discussed in cotangent graphs. It is starting from the maximum of the sine graph and only displaying one half of it. It is there because the ratios on the unit circle dictate that it is positive and that it must avoid the zero asymptote. This is one portion of the graph though. Between the asymptotes there are two halves. the other half lies in the second quadrant.
(https://www.desmos.com/calculator/hjts26gwst)
In the second quadrant we see a full parabola. The graph remains positive still because the unit circle dictates that it will remain positive. The second portion of the parabola starts at the maximum of the sine graph as well. Now we have a positive parabola that originates off of sine's maximum and avoids the two quadrant angles that create an undefined value (the asymptotes).
In the third quadrant we see the cosecant graph turn negative just like the sine graph (because they are the same values just in different positions in the ratio) and it starts a negative parabola. It touches at the minimum of the sine graph and turns downwards. It avoids the asymptote on the left because it is still an undefined value dictated by the unit circle values of y. This is again only a piece of it.
The fourth quadrant is also negative because of the unit circle values that are present in the ratio. It also starts from the minimum of the sine graph and turns right down and avoids the 2pi asymptote that is created by the y being a negative number. This forms the last part of the parabola. Now we have our full period for a cosecant graph.
Secant
When we are looking at our secant graphs they will have the same asymptotes as the tangent graph because both have x on the bottom and that means that they will have undefined ratios in the exact same spots. This graph will be avoiding those spots and will follow the cosine graph in the positive and negative areas of the quadrants on the graph (for that is what is dictated by the unit circle). It is not too difficult to understand, but you will need your cosine graph in order to create the secant graph.
(https://www.desmos.com/calculator/hjts26gwst)
In the picture we see that the first part of the graph is positive like the cosine graph and that is because of the values that are given in the unit circle. Then we see that it is coming off the maximum of the cosine graph. We notice that it is avoiding the asymptote because that is a zero on the bottom of the ratio and that is not allowed. This is only part of the parabola that it forms. The other one is in the opposite revolution of the circle so it is not shown there, but it is there and it avoids the asymptote on that side of it. It is all pretty simple.
In the second photo we see that the graph avoids the asymptote that is not allowed to touch since that is a zero in the denominator (cosine). Then we notice that it is coming off of the minimum of the cosine graph because they contain the same x values since it is the unit circle that we base the parent graph off of. It is not too difficult to understand. It will have another half to make the complete parabola and that lies in the third quadrant.
(https://www.desmos.com/calculator/hjts26gwst) In the third quadrant (which is also negative due to the ratio values that are present) it forms the other half of the parabola. It stems off the minimum of the cosine graph. It curves down since it is negative and goes all the way to almost the next angle (on the unit circle) but avoids it for it is a zero value making it undefined and an asymptote. This gives it the parabola shape unlike the original graph.
(https://www.desmos.com/calculator/hjts26gwst)
In the fourth quadrant we see that it is positive because the unit circle ratios make it positive. It does need to avoid the asymptote on its left though because that is an undefined ratio so it does not exist. It curves and sprouts from the maximum of the cosine graph and starts the parabola shape. It makes it the parabola shape that it needs to have. This is a whole period even though it does not look like it. It is.
Overall
In the end we see that the asymptotes that the rest of these graphs have are the reasons that they are shaped differently and all of them have something in common with the original graphs of sine and cosine.
Resources.
Mrs. Kirch on the wonderful website of https://www.desmos.com/calculator/hjts26gwst
Thursday, April 17, 2014
BQ#5 – Unit T Concepts 1-3
No Asymptotes for Sine or Cosine? Oh my!
Now you might be thinking, what? why are there no asymptotes for sine and cosine, but asymptotes for everything else? Well the answer is pretty simple. All we need to do is take a look at the ratios for each trig function according to the unit circle.
Now you might be thinking, what? why are there no asymptotes for sine and cosine, but asymptotes for everything else? Well the answer is pretty simple. All we need to do is take a look at the ratios for each trig function according to the unit circle.
When we look at the ratios we see that sine and cosine of theta are divided by r while everything else is divided by x or y. Now we know that on the unit circle r is equal to one so that means that sine and cosine will be divided by one. An asymptote is defined as being an undefined ratio. Since sine and cosine will never be divided by a zero they will never have an asymptote. Everything else will. Now tangent and secant will have the same asymptotes because they are both divided by x and then cosecant and cotangent will have the same asymptotes because they are both divided by y. The whole thing is as easy as r-x-y.
Reference
Wednesday, April 16, 2014
BQ#2 – Unit T Concept Intro
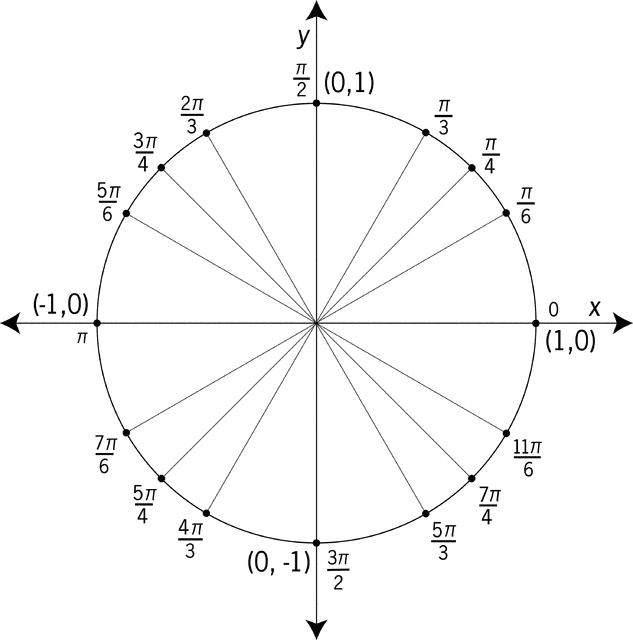 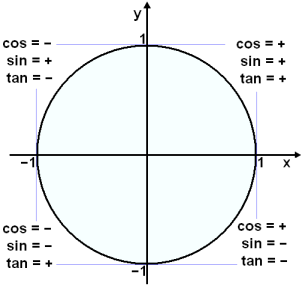 |
| (http://etc.usf.edu/clipart/43200/43204/unit-circle12_43204_md.gif http://www.rasmus.is/uk/t/F/Su36k02_m06.gif)
This is an image of the unit circle in radians since when we graph sine, cosine, tangent, and cotangent it is easier to graph them in radians than in degrees. It is more of a challenge to see the graph on the calculator when we use degrees. That is why the radians are used. Now we go on to explain why the periods for the different graphs are the way they are. In reality they all correspond to the unit circle.
The second image is a picture of the positive and negative ratios in each quadrant. This will also help understand the graphs.
Sine
When we look at the unit circle for sine we see that it goes positive in the first quadrant, positive in the second quadrant, negative in the third quadrant, and negative in the fourth quadrant. Now if we take the unit circle and stretch it out on a graph we see that it works like this.
With this graph it is positive in the first quadrant (0-pi/2) and then also positive in the second quadrant (pi/2-pi). Then it drops below the x axis and becomes negative in quadrant three (pi-3pi/2) and quadrant four (3pi/2-2pi). This is known as a pattern and will continue on forever in both directions. This repetition makes it cyclical. The period is the amount of time it takes for it to get through one cycle on the graph. That means that the period for the sine graph is 2pi. This is because when before we repeat the pattern of positive positive negative negative we go through the whole unit circle which is two pi. That means we will continually see the start of a new cycle after every two pi. They cover 2pi on the graph before restarting basically. This all happens due to the y values on the unit circle.
Cosine
When we are looking at cosine it goes positive in the first quadrant, negative in the second quadrant, negative in the third quadrant, positive in the fourth quadrant on the unit circle. When we stretch the unit circle out we get this on our graph also.

As we look at the graph we see that it matches the unit circle. It is positive in the first quadrant (1-pi/2), negative in the second (pi/2-pi), negative in the third quadrant (pi-3pi/2) and positive in the fourth quadrant (3pi/2-2pi). That shows the unit circle on a graph. It matches the x values on the unit circle. The unit circle dictates it. The period for this cyclical graph is also 2pi. That is because it takes that long to get to a repetition. Then the cycles can start again. They always match the circle and the graph. This will go on continuously forever.
The graph below shows sine and cosine overlapping on the same graph to just give visuals.
Tangent/Cotangent
When we look at the unit circle we see that the first quadrant is positive, the second negative, the third positive, and the fourth negative on the unit circle. If you do this to the graph you will see that this is the same thing.
This graph shows the quadrants on the graph along with the asymptotes. The asymptotes are at pi/2 and at 3pi/2. When we look at the graph we see that it is positive (0- little before pi/2), then negative (little after pi/2-pi), then positive (pi-little before 3pi/2), and negative (little after 3pi/2-2pi). They do not touch pi/2 or 3pi/2 because those are asymptotes and cannot be touched. The asymptote is there because the value is undefined. The reason that the period is only pi for this one is because it takes one pi to start a repetition of the cycle. It is all that simple.
Amplitude
The reason that sine and cosine have an amplitude is because they are the only ones with restrictions. This is because the ratio is y/r and x/r so they can only be as far as the one on the parent graphs. They can only reach the 1 on the top and the -1 on the bottom. The other ratios have no amplitudes because they have no restrictions. It is as easy as that.
References http://etc.usf.edu/clipart/43200/43204/unit-circle12_43204_md.gif http://www.rasmus.is/uk/t/F/Su36k02_m06.gif |
Wednesday, April 2, 2014
Reflection #1 - Unit Q: Verifying Trig Identities
Reflection:
1. To verify a trig function really means that you are checking to make sure that whatever the equation says is true. You are basically solving the trig function and if all goes well it should be the same single trig function that is on the other side of the equal side. To verify means to go through the problem step by step and use the identities to replace functions and cancel out other functions and in the end (a majority of the time) get a single trig function. If you worked each step right and paid attention to all the details the left and right side of the equations should match up. To verify means to check and that is what you are doing. It is like when you are plugging x back into an equation, you are verifying (checking) to make sure that it is true. Try not to complicate it. You are reducing, replacing, and canceling out trig functions on the left side of the equal sign to make sure it matches the right side.
2. As I have gone through this unit I have found that it is always easy to take some deep breaths and then begin in on the problem. You always have to try, even if you think that it is too hard and you cannot do it, you still need to try. Sometimes when you try you can get the answer or a lead in the right direction. It is always good to try. You will never succeed if you don't try, if all you can say is you can't. Another thing that I find helpful is to try to make it so that the answer is in terms that you understand or make things into identities to shrink the problem down. Always make it smaller. It can help you understand it, to take it in and relax. Also try and get rid of the fractions on fractions. This can lead to ratios canceling out and your life getting a little bit easier. Always try to make the problem something that you can look at and understand. I always find it easier when you can break the problem up and just look at it section by section and then look at the big picture. It is always easier to make the problem a little bit more manageable Remember that it is always better to try and fail than to never try at all. Oh one more thing. Never forget your theta or x or whatever the variable is (they are very important to the overall understanding of the problem). Good luck.
3. When I first see a problem I like to take a breath and see what type of problem it is (verification or simplifying or solving). From there I take it step by step. I see if I can make anything cancel out easily. If that does not work and I feel like I am stuck I change the trig functions to sin and cos because it is easier to work with. With that it is easier to try and figure things out. We then have the ability to factor or multiply. With that it is just easier to go about working with it. If it is a large one, then we just have to look at it in pieces. Make it so that it is easy to work with. I don't really know if my thought process is clear, but I just look and see the best route. The best replacements and cancelations. It is easier for me to work things out when they are smaller and a little broken down. It is easier to take it all in when it is in chunks. I just look for all the simple ways to get it done. That is my plan of action. Not the clearest or the best, but it works for me.
1. To verify a trig function really means that you are checking to make sure that whatever the equation says is true. You are basically solving the trig function and if all goes well it should be the same single trig function that is on the other side of the equal side. To verify means to go through the problem step by step and use the identities to replace functions and cancel out other functions and in the end (a majority of the time) get a single trig function. If you worked each step right and paid attention to all the details the left and right side of the equations should match up. To verify means to check and that is what you are doing. It is like when you are plugging x back into an equation, you are verifying (checking) to make sure that it is true. Try not to complicate it. You are reducing, replacing, and canceling out trig functions on the left side of the equal sign to make sure it matches the right side.
2. As I have gone through this unit I have found that it is always easy to take some deep breaths and then begin in on the problem. You always have to try, even if you think that it is too hard and you cannot do it, you still need to try. Sometimes when you try you can get the answer or a lead in the right direction. It is always good to try. You will never succeed if you don't try, if all you can say is you can't. Another thing that I find helpful is to try to make it so that the answer is in terms that you understand or make things into identities to shrink the problem down. Always make it smaller. It can help you understand it, to take it in and relax. Also try and get rid of the fractions on fractions. This can lead to ratios canceling out and your life getting a little bit easier. Always try to make the problem something that you can look at and understand. I always find it easier when you can break the problem up and just look at it section by section and then look at the big picture. It is always easier to make the problem a little bit more manageable Remember that it is always better to try and fail than to never try at all. Oh one more thing. Never forget your theta or x or whatever the variable is (they are very important to the overall understanding of the problem). Good luck.
3. When I first see a problem I like to take a breath and see what type of problem it is (verification or simplifying or solving). From there I take it step by step. I see if I can make anything cancel out easily. If that does not work and I feel like I am stuck I change the trig functions to sin and cos because it is easier to work with. With that it is easier to try and figure things out. We then have the ability to factor or multiply. With that it is just easier to go about working with it. If it is a large one, then we just have to look at it in pieces. Make it so that it is easy to work with. I don't really know if my thought process is clear, but I just look and see the best route. The best replacements and cancelations. It is easier for me to work things out when they are smaller and a little broken down. It is easier to take it all in when it is in chunks. I just look for all the simple ways to get it done. That is my plan of action. Not the clearest or the best, but it works for me.
Wednesday, March 26, 2014
SP7: Unit Q Concept 2: Finding all six trig functions using trigonometric identities.
This SP7 was made in collaboration with Miguel G. Please visit the other awesome posts on their blog by going here.
In this post we will be finding the six different trigonometric ratios using two different routes. The first is for this current concept-using the identities- and the second for a concept in unit o- using the triangle-, however your answers will be the same exact thing. Be careful to pay attention as we walk you through the problem step by step and each identity. It is simple and easy to understand once you get a walk through. Here we go.
Unit Q Concept 2
Unit O Concept 5
As you can tell they are the same for both of the concepts. The answers are the same, the path to that answer is different. Both ways will work though.
Subscribe to:
Posts (Atom)


.png)









.png)
.png)
.png)
.png)
.png)
.png)


.png)
.png)
.png)
.png)
.png)

.png)
.png)














